- Home
- Study Guides
Surd is a number that cannot be expressed as a fraction, or whose fractional representation has an integer and a fractional part. The most famous surd is the square root of $2$, which is approximately $1.4142135623730950488016887242096980785696$… We also use surds to express irrational numbers accurately as we cannot write them in decimal form – the decimals of irrational numbers recur or do not stop.
Surds are often represented by square roots $(\sqrt{\square })$, cube roots $(\sqrt[3]{\square })$ and other root symbols.
Laws of Surds
To solve problems involving surds, we must first understand the two rules of surds.
- $\sqrt{ab}=\sqrt{a}\times \sqrt{b}$
- $\sqrt{\frac{a}{b}}=\frac{\sqrt{a}}{\sqrt{b}}$
Practice Question 1
Simplify each of the following surds without using a calculator.
(i)
$\sqrt{40}$
(i) $\sqrt{40}$
(ii)
$\sqrt{18}$
(ii) $\sqrt{18}$
(iii)
$\sqrt{396}$
(iii) $\sqrt{396}$
- (i)
- (ii)
- (iii)
$40=4\times 10$
$\begin{aligned}
\sqrt{40}&=\sqrt{4\times 10} \\
& =\sqrt{4}\times \sqrt{10} \\
& =2\sqrt{10}
\end{aligned}$
$18=9\times 2$
$\begin{aligned}
\sqrt{18}&=\sqrt{9\times 2} \\
& =\sqrt{9}\sqrt{2} \\
& =3\sqrt{2}
\end{aligned}$
$\begin{aligned}
\sqrt{396}&=\sqrt{4\times 9\times 11} \\
& =\sqrt{4}\sqrt{9}\sqrt{11} \\
& =2\times 3\sqrt{11} \\
& =6\sqrt{11}
\end{aligned}$
- (i)
- (ii)
- (iii)
$40=4\times 10$
$\begin{aligned}
\sqrt{40}&=\sqrt{4\times 10} \\
& =\sqrt{4}\times \sqrt{10} \\
& =2\sqrt{10}
\end{aligned}$
$18=9\times 2$
$\begin{aligned}
\sqrt{18}&=\sqrt{9\times 2} \\
& =\sqrt{9}\sqrt{2} \\
& =3\sqrt{2}
\end{aligned}$
$\begin{aligned}
\sqrt{396}&=\sqrt{4\times 9\times 11} \\
& =\sqrt{4}\sqrt{9}\sqrt{11} \\
& =2\times 3\sqrt{11} \\
& =6\sqrt{11}
\end{aligned}$
Addition and Subtraction of Surds
To perform addition or subtraction on surds, we must make sure that the numbers within the square roots are identical. For instance,
$\begin{aligned}
& 3\sqrt{2}+5\sqrt{2} \\
& 11\sqrt{3}-4\sqrt{3} \\
\end{aligned}$
Practice Question 2
Simplify each of the following surds without using a calculator.
(i)
$\sqrt{75}+\sqrt{108}$
(i) $\sqrt{75}+\sqrt{108}$
(ii)
$\sqrt{32}+\sqrt{50}$
(ii) $\sqrt{32}+\sqrt{50}$
(iii)
$\left( 3+5\sqrt{2} \right)\left( 4-\sqrt{2} \right)$
(iii) $\left( 3+5\sqrt{2} \right)\left( 4-\sqrt{2} \right)$
- (i)
- (ii)
- (iii)
$\begin{aligned} &\sqrt{75}+\sqrt{108}\\
& =\sqrt{25\times 3}+\sqrt{9\times 4\times 3} \\
& =\sqrt{25}\sqrt{3}+\sqrt{9}\sqrt{4}\sqrt{3} \\
& =5\sqrt{3}+3\cdot 2\sqrt{3} \\
& =5\sqrt{3}+6\sqrt{3} \\
& =11\sqrt{3} \\
\end{aligned}$
$\begin{aligned}
& \sqrt{32}+\sqrt{50} \\
& =\sqrt{16\cdot 2}+\sqrt{25\cdot 2} \\
& =\sqrt{16}\sqrt{2}+\sqrt{25}\sqrt{2} \\
& =4\sqrt{2}+5\sqrt{2} \\
& =9\sqrt{2}
\end{aligned}$
$\begin{aligned}
& \left( 3+5\sqrt{2} \right)\left( 4-\sqrt{2} \right) \\
& =12-3\sqrt{2}+20\sqrt{2}+5\sqrt{2}\left( -\sqrt{2} \right) \\
& =12+17\sqrt{2}-5\left( 2 \right) \\
& =12-10+17\sqrt{2} \\
& =2+17\sqrt{2} \\
\end{aligned}$
- (i)
- (ii)
- (iii)
$\begin{aligned} &\sqrt{75}+\sqrt{108}\\
& =\sqrt{25\times 3}+\sqrt{9\times 4\times 3} \\
& =\sqrt{25}\sqrt{3}+\sqrt{9}\sqrt{4}\sqrt{3} \\
& =5\sqrt{3}+3\cdot 2\sqrt{3} \\
& =5\sqrt{3}+6\sqrt{3} \\
& =11\sqrt{3} \\
\end{aligned}$
$\begin{aligned}
& \sqrt{32}+\sqrt{50} \\
& =\sqrt{16\cdot 2}+\sqrt{25\cdot 2} \\
& =\sqrt{16}\sqrt{2}+\sqrt{25}\sqrt{2} \\
& =4\sqrt{2}+5\sqrt{2} \\
& =9\sqrt{2}
\end{aligned}$
$\begin{aligned}
& \left( 3+5\sqrt{2} \right)\left( 4-\sqrt{2} \right) \\
& =12-3\sqrt{2}+20\sqrt{2}+5\sqrt{2}\left( -\sqrt{2} \right) \\
& =12+17\sqrt{2}-5\left( 2 \right) \\
& =12-10+17\sqrt{2} \\
& =2+17\sqrt{2} \\
\end{aligned}$
Conjugate Surds
Conjugate surds, also known as complementary surds, are formed when we change the sign between the two terms. Broadly speaking, conjugate surds refer to the sum and difference of two simple quadratic surds – e.g. $5+\sqrt{3}$ and $5-\sqrt{3}$.
What happens when we multiply the surd and its conjugate together? We will get a rational number.
$\left( 5+\sqrt{3} \right)\left( 5-\sqrt{3} \right)={{5}^{2}}-3=22$
Practice Question 3
Simplify each of the following surds without using a calculator.
(i)
$\frac{2}{\sqrt{5}-3}$
(i) $\frac{2}{\sqrt{5}-3}$
(ii)
$\frac{10}{\sqrt{2}-5}$
(ii) $\frac{10}{\sqrt{2}-5}$
(iii)
$\frac{8}{3\sqrt{7}-1}$
(iii) $\frac{8}{3\sqrt{7}-1}$
- (i)
- (ii)
- (iii)
$\begin{aligned}
& \frac{2}{\sqrt{5}-3}\cdot \frac{\left( \sqrt{5}+3 \right)}{\left( \sqrt{5}+3 \right)} \\
& =\frac{2\left( \sqrt{5}+3 \right)}{{{\left( \sqrt{5} \right)}^{2}}-{{\left( 3 \right)}^{2}}} \\
& =\frac{2\sqrt{5}+6}{5-9} \\
& =\frac{2\sqrt{5}+6}{-4} \\
& =-\left[ \frac{2\sqrt{5}}{4}+\frac{6}{4} \right] \\
& =-\left( \frac{1}{2}\sqrt{5}+\frac{3}{2} \right) \\
\end{aligned}$
$\begin{aligned}
& \frac{10}{\sqrt{2}-5}\cdot \frac{\left( \sqrt{2}+5 \right)}{\left( \sqrt{2}+5 \right)} \\
& =\frac{10\sqrt{2}+10\cdot 5}{{{\left( \sqrt{2} \right)}^{2}}+{{\left( 5 \right)}^{2}}} \\
& =\frac{10\sqrt{2}+50}{2-25} \\
& =\frac{10\sqrt{2}+50}{-23} \\
& =-\frac{10\sqrt{2}+50}{23} \\
\end{aligned}$
$\begin{aligned}
& \frac{8}{3\sqrt{7}-1}\cdot \frac{\left( 3\sqrt{7}+1 \right)}{\left( 3\sqrt{7}+1 \right)} \\
& =\frac{24\sqrt{7}+8}{{{\left( 3\sqrt{7} \right)}^{2}}-{{\left( 1 \right)}^{2}}} \\
& =\frac{24\sqrt{7}+8}{63-1} \\
& =\frac{24\sqrt{7}+8}{62} \\
& =\frac{24}{62}\sqrt{7}+\frac{8}{62} \\
& =\frac{12}{31}\sqrt{7}+\frac{4}{31} \\
\end{aligned}$
- (i)
- (ii)
- (iii)
$\begin{aligned}
& \frac{2}{\sqrt{5}-3}\cdot \frac{\left( \sqrt{5}+3 \right)}{\left( \sqrt{5}+3 \right)} \\
& =\frac{2\left( \sqrt{5}+3 \right)}{{{\left( \sqrt{5} \right)}^{2}}-{{\left( 3 \right)}^{2}}} \\
& =\frac{2\sqrt{5}+6}{5-9} \\
& =\frac{2\sqrt{5}+6}{-4} \\
& =-\left[ \frac{2\sqrt{5}}{4}+\frac{6}{4} \right] \\
& =-\left( \frac{1}{2}\sqrt{5}+\frac{3}{2} \right) \\
\end{aligned}$
$\begin{aligned}
& \frac{10}{\sqrt{2}-5}\cdot \frac{\left( \sqrt{2}+5 \right)}{\left( \sqrt{2}+5 \right)} \\
& =\frac{10\sqrt{2}+10\cdot 5}{{{\left( \sqrt{2} \right)}^{2}}+{{\left( 5 \right)}^{2}}} \\
& =\frac{10\sqrt{2}+50}{2-25} \\
& =\frac{10\sqrt{2}+50}{-23} \\
& =-\frac{10\sqrt{2}+50}{23} \\
\end{aligned}$
$\begin{aligned}
& \frac{8}{3\sqrt{7}-1}\cdot \frac{\left( 3\sqrt{7}+1 \right)}{\left( 3\sqrt{7}+1 \right)} \\
& =\frac{24\sqrt{7}+8}{{{\left( 3\sqrt{7} \right)}^{2}}-{{\left( 1 \right)}^{2}}} \\
& =\frac{24\sqrt{7}+8}{63-1} \\
& =\frac{24\sqrt{7}+8}{62} \\
& =\frac{24}{62}\sqrt{7}+\frac{8}{62} \\
& =\frac{12}{31}\sqrt{7}+\frac{4}{31} \\
\end{aligned}$
Equation involving Surds
In this section, we will learn how to find the solution of equations involving surds.
Practice Question 4
It is given that $a$ and $b$ are positive integers such that $\left( a\sqrt{5}-1 \right)\left( \sqrt{5}+b \right)=20\sqrt{5}+32$. Form a pair of simultaneous equations and solve them to find the value of $a$ and of $b$.
- Solution
$\begin{aligned}
\left( a\sqrt{5}-1 \right)\left( \sqrt{5}+b \right)&=20\sqrt{5}+32 \\
a\sqrt{5}\sqrt{5}+ab\sqrt{5}-\sqrt{5}-b&=20\sqrt{5}+32 \\
\left( 5a-b \right)+\sqrt{5}\left( ab-1 \right)&=32+20\sqrt{5}
\end{aligned}$
By comparing,
$5a-b=32—(2)$,
$\begin{aligned}
ab-1&=20 \\
ab&=21 \\
a&=\frac{21}{b}—(1)
\end{aligned}$
Substitute $(1)$ into $(2)$:
$\begin{aligned}
5\left( \frac{21}{b} \right)-b&=32 \\
\frac{105}{b}-b&=32 \\
105-{{b}^{2}}&=32b \\
{{b}^{2}}+32b-105&=0 \\
\left( b-3 \right)\left( b+35 \right)&=0
\end{aligned}$
$\therefore b=3$ or $-35$
When $b=3,a=\frac{21}{3}=7$
When $b=-35,a=-\frac{35}{3}$ (rej, as $a$ and $b$ are positive)
$\therefore a=7,b=3$
Practice Question 5
Solve $\sqrt{7x-5}-x-1=0$.
- Solution
$\begin{aligned}
\sqrt{7x-5}&=x+1 \\
{{\left( \sqrt{7x-5} \right)}^{2}}&={{\left( x+1 \right)}^{2}} \\
7x-5&={{x}^{2}}+2x+1 \\
0&={{x}^{2}}-5x+6 \\
0&=\left( x-3 \right)\left( x-2 \right)\\
x&=2 \text{ or } 3\\\end{aligned}$
When $x=2$,
$\begin{aligned}
\text{LHS}&=\sqrt{7\left( 2 \right)-5}-2-1 \\
& =0 \\
& =\text{RHS}
\end{aligned}$
When $x=3$,
$\begin{aligned}
\text{LHS}&=\sqrt{7\left( 3 \right)-5}-3-1 \\
& =0 \\
& =\text{RHS}
\end{aligned}$
$\therefore x=2$ or $3$
Applications of Surds
One interesting consequence of using surds in order to eliminate errors is that the use of the surds implies the use of infinitely small quantities. In physics, surds are often employed to make sure that important calculations are precise. Surds are also critical in engineering, for instance, in constructing bridges, which need to be structurally sturdy.
Practice Question 6
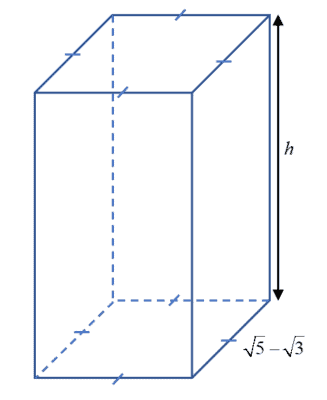
A rectangular block has a square base. The length of each side of the base is $\left( \sqrt{5}-\sqrt{3} \right)$m and the volume of the block is $\left( 4\sqrt{3}-2\sqrt{5} \right)$ m3. Find, without the use of a calculator, the height of the block in the form of $a\sqrt{3}+b\sqrt{5}$.
- Diagram
- Solution
Let height be $h$,
Volume$={{\left( \sqrt{5}-\sqrt{3} \right)}^{2}}h$
$\begin{aligned}
4\sqrt{3}-2\sqrt{5}&={{\left( \sqrt{5}-\sqrt{3} \right)}^{2}}h \\
h&=\frac{4\sqrt{3}-2\sqrt{5}}{{{\left( \sqrt{5}-\sqrt{3} \right)}^{2}}} \\
& =\frac{4\sqrt{3}-2\sqrt{5}}{{{\left( \sqrt{5} \right)}^{2}}-2\sqrt{5}\sqrt{3}+{{\left( \sqrt{3} \right)}^{2}}} \\
& =\frac{4\sqrt{3}-2\sqrt{5}}{5-2\sqrt{5\times 3}+3} \\
& =\frac{2\left( 2\sqrt{3}-\sqrt{5} \right)}{8-2\sqrt{15}} \\
& =\frac{2\left( 2\sqrt{3}-\sqrt{5} \right)}{2\left( 4-\sqrt{15} \right)} \\
& =\frac{2\left( 2\sqrt{3}-\sqrt{5} \right)}{2\left( 4-\sqrt{15} \right)}\cdot \frac{\left( 4+\sqrt{15} \right)}{\left( 4+\sqrt{15} \right)} \\
& =\frac{8\sqrt{3}+2\sqrt{3}\sqrt{15}-4\sqrt{5}-\sqrt{5}\sqrt{15}}{{{\left( 4 \right)}^{2}}-{{\sqrt{15}}^{2}}} \\
& =\frac{8\sqrt{3}+2\sqrt{3}\sqrt{3}\sqrt{5}-4\sqrt{5}-\sqrt{5}\sqrt{3}\sqrt{5}}{16-15} \\
& =8\sqrt{3}+2\left( 3 \right)\sqrt{5}-4\sqrt{5}-5\sqrt{3} \\
& =8\sqrt{3}+6\sqrt{5}-4\sqrt{5}-5\sqrt{3} \\
& =3\sqrt{3}+2\sqrt{5}
\end{aligned}$
Practice Question 7
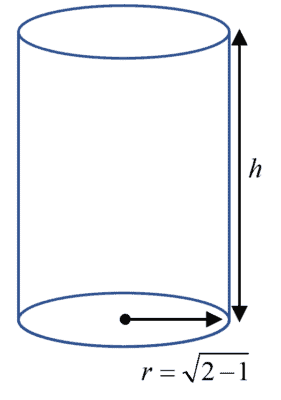
A cylinder has a radius of $\left( \sqrt{2}-1 \right)$cm and a volume of $\left( 12+4\sqrt{2} \right)\pi$ cm3. Find, without using a calculator, the exact value of its height, $h$, in the form $\frac{a+b\sqrt{2}}{c}$cm, where $a$, $b$ and $c$ are integers.
- Diagram
- Solution
Volume of cylinder$=\pi {{r}^{2}}h$
Volume$=\left( 12+4\sqrt{2} \right)\pi $
$\begin{aligned}
\pi {{\left( \sqrt{2}-1 \right)}^{2}}h&=\left( 12+4\sqrt{2} \right)\pi \\
{{\left( \sqrt{2}-1 \right)}^{2}}h&=12+4\sqrt{2} \\
h&=\frac{12+4\sqrt{2}}{{{\left( \sqrt{2}-1 \right)}^{2}}} \\
& =\frac{4\left( 3+\sqrt{2} \right)}{{{\sqrt{2}}^{2}}-2\left( \sqrt{2} \right)+1} \\
& =\frac{4\left( 3+\sqrt{2} \right)}{\left( 3-2\sqrt{2} \right)} \\
& =\frac{4\left( 3+\sqrt{2} \right)}{\left( 3-2\sqrt{2} \right)}\cdot \frac{\left( 3+2\sqrt{2} \right)}{\left( 3+2\sqrt{2} \right)} \\
& =\frac{4\left( 9+6\sqrt{2}+3\sqrt{2}+2\sqrt{2}\sqrt{2} \right)}{{{\left( 3 \right)}^{2}}-{{\left( 2\sqrt{2} \right)}^{2}}} \\
& =\frac{4\left( 9+9\sqrt{2}+4 \right)}{9-4\left( 2 \right)} \\
& =\frac{4\left( 13+9\sqrt{2} \right)}{1} \\
& =\frac{52+36\sqrt{2}}{1} \\
& =52+36\sqrt{2}\,\text{cm}
\end{aligned}$
Do sign up for our Free Mini-Courses and try our well-structured curriculum to see how it can help to maximize your learning in mathematics online.
Secondary School Additional Mathematics Free Online Course Sign Up.
You may also find the pricing and plans for our Additional Mathematics unlimited all-access courses here!
Topic List
Free Mini Course
With our online courses, you can learn at your own pace and take your time whenever it’s most convenient for you. Experience learning with us now for free!
Topics included:
- Derivative as a Gradient Function
- Chain Rule
- Problems involving Tangents and Normals
- Connected Rate of Change Involving Area